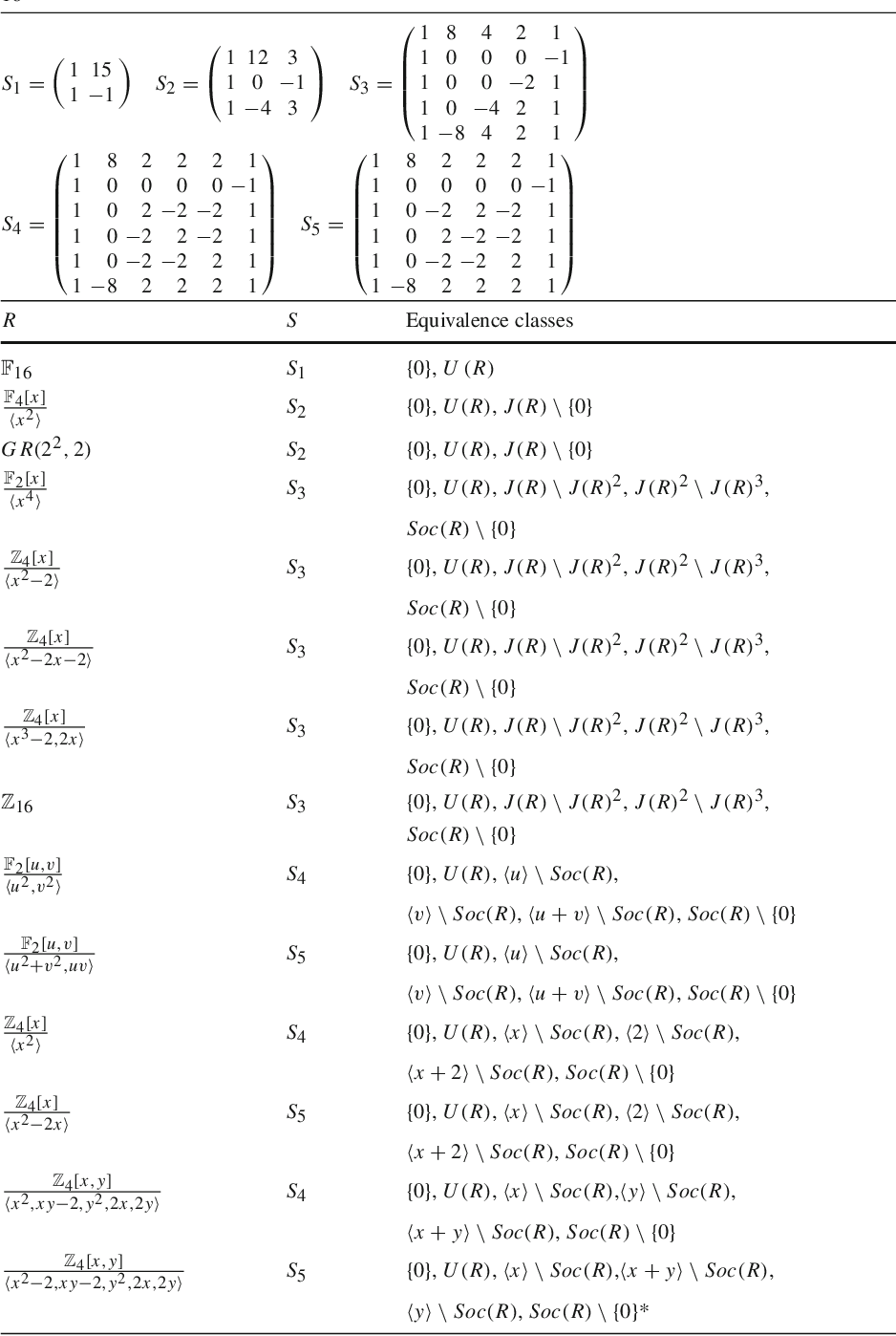
2) ASSE2 Use the structure of an expression to identify ways to rewrite it For example, see x4 – y 4 as (x2)2 – (y2)2, thus recognizing it as a difference of squares that can be factored as (x2 – y 2)(x2 y2) 3) ASS Choose and produce an equivalent form of an expression to reveal and explain propertiesFor example, the polynomial identity (x 2 y 2) 2 = (x 2 – y 2) 2 (2xy) 2 can be used to generate Pythagorean triples Suggested Learning Targets Understand that polynomial identities include but are not limited to the product of the sum and difference of two terms, the difference of two squares, the sum and difference of two cubes, the4 Prove polynomial identities and use them to describe numerical relationships For example, the polynomial identity (x 2 y 2) 2 = (x 2 – y 2) 2 (2xy) 2 can be used to generate Pythagorean triples With the increase in technology and this huge new thing called the Internet, identity theft has become a worldwide problem

Polar Coordinates
The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate
The identity (x^2 y^2)^2=(x^2-y^2)^2 (2xy)^2 can be used to generate-Understand that the zero and identity matrices play a role in matrix addition and multiplication similar to the role of 0 and 1 in the real numbers (x^2 y^2)^2 = (x^2 y^2)^2 (2xy)^2 can be used to generate Pythagorean triples AAPR5 Know and apply that the Binomial Theorem gives the expansion of (x y)^n in powers of x and y for aFor example, the polynomial identity (x² y²)² = (x² – y²)² (2xy)² can be used to generate Pythagorean triples Lesson/Activity Lesson/Activity Description Suggested Technology Special Products of Binomials MAFS912AAPR11 In this video, students will
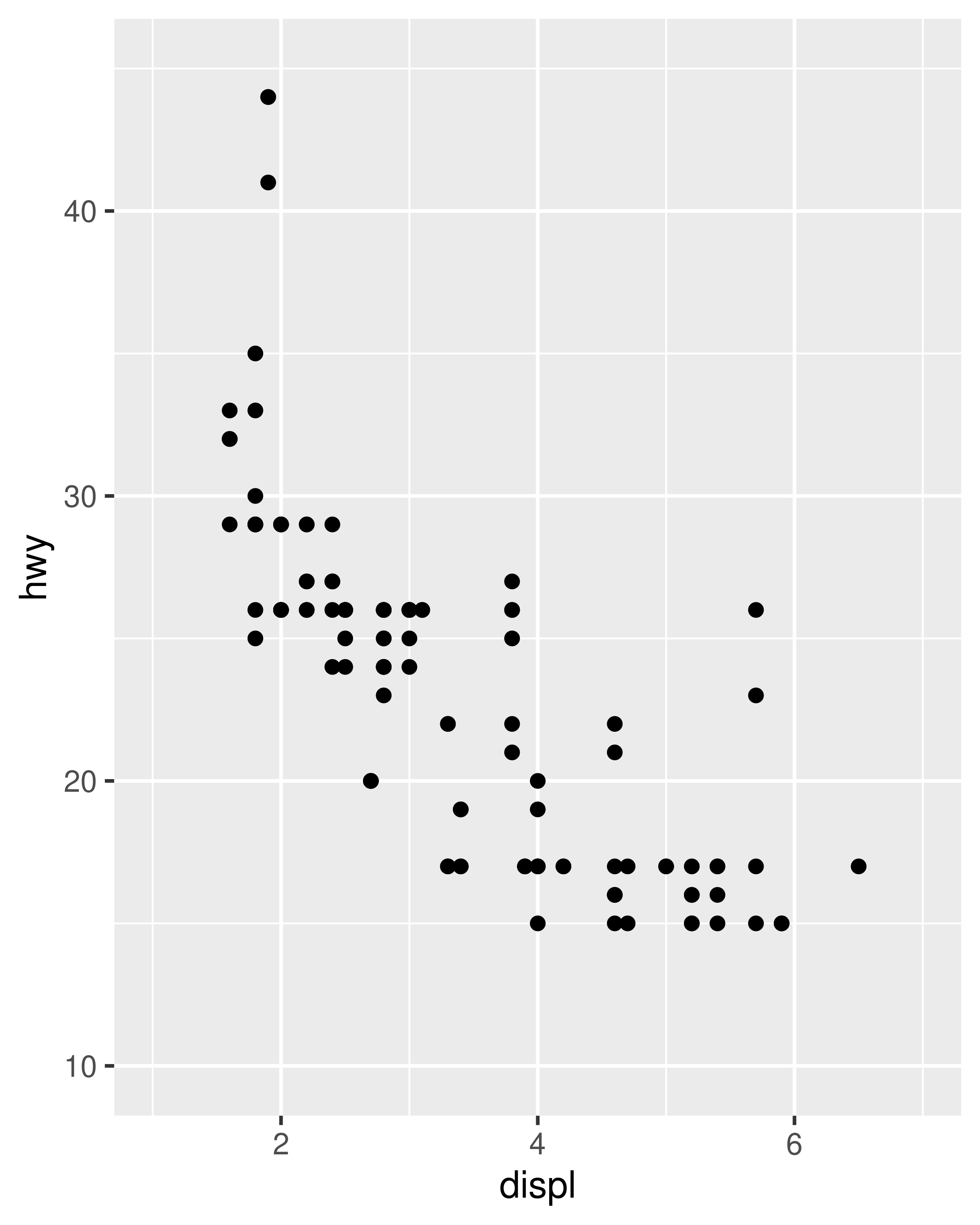



10 Position Scales And Axes Ggplot2
Example, the polynomial identity (x2y2)2 = (x2– y2)2 (2xy)2 can be used to generate Pythagorean triples SE/TE Concept Byte 318 Algebra Arithmetic with Polynomials and Rational Expressions (AAPR) Rewrite rational expressions HSAAPRD6 Rewrite simple rational expressions in different forms; The difference of squares identity can be written a2 −b2 = (a −b)(a b) We will use this below with a = (4x −3) and b = 1 I prefer not to have to do much arithmetic involving fractions, so I would premultiply this equation by 8 to avoid them and get 0 = 8(2x2 − 3x 1) 0 = 16x2 − 24x 8 0 = 16x2 − 24x 9 − 1 0 = (4x − 3)2For example, the polynomial identity (x2 y2)2 = (x2 y2)2 (2xy)2 can be used to generate Pythagorean triples Rewrite rational expressions Integrated Math II Clarification Linear and quadratic denominators
AAPR4 Prove polynomial identities and use them to describe numerical relationships For example, the polynomial identity (x2 y2)2 = (x2 y2)2 (2xy)2 can be used to generate Pythagorean triples Rewrite rational expressionsThe quadratic formula gives two solutions, one when ± is addition and one when it is subtraction y^ {2}2xyx^ {2}=0 y 2 2 x y x 2 = 0 This equation is in standard form ax^ {2}bxc=0 Substitute 1 for a, 2x for b, and x^ {2} for c in the quadratic formula, \frac {b±\sqrt {b^ {2 Give possible expressions for the length and breadth of each of the following rectangles, in which their areas are given (i) Area 25a2 – 35a 12 (ii) Area 35y2 13y – 12 Solution (i) We have, area of rectangle = 25a 2 – 35a12 = 25a 2 – a – 15a12
Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!29 P a g e ( R e v A u g u s t 2 0 1 8 ) D Represent and solve equations and inequalities graphically 11 Explain why the xcoordinates of the points where the graphs of the equations y = f(x) and y = g(x) intersect are the solutions of the equation f(x) = g(x);Select two answers one for x and one for y 15 10
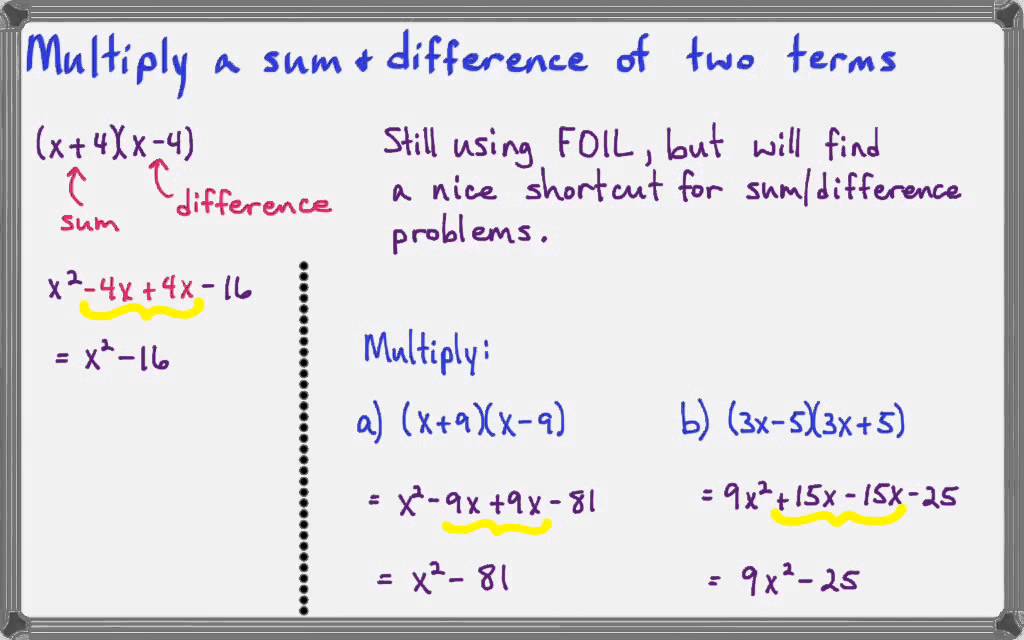



Polynomial Identities Solutions Examples Videos Worksheets Games Activities




Solved Find The Volume Of The Solid Whose Base Is The Region Chegg Com
Yes, sec 2 x−1=tan 2 x is an identity sec 2 −1=tan 2 x Let us derive the equation We know the identity sin 2 (x)cos 2 (x)=1 ——(i) Dividing throughout the equation by cos 2 (x) We get sin 2 (x)/cos 2 (x) cos 2 (x)/cos 2 (x) = 1/cos 2 (x) We know that sin 2 (x)/cos 2 (x)= tan 2 (x), and cos 2 (x)/cos 2 (x) = 1 So the equation (i) after substituting becomes Identities V Last updated at by Teachoo Identity V is (a b c) 2 = a 2 b 2 c 2 2ab 2bc 2ca Let us prove it Proof (a b c) 2 = ( (a b) c) 2 Using (x y) 2 = x 2 y 2 2xyFor example, the polynomial identity (x^2 y^2)^2 = (x^2 – y^2)^2 (2xy)^2 can be used to generate Pythagorean triples 5 () Know and apply the Binomial Theorem for the expansion of (x y)^n in powers of x and y for a positive integer n, where x and y are any numbers, with coefficients determined for example by Pascal's Triangle1




Pythagorean Triple Wikipedia
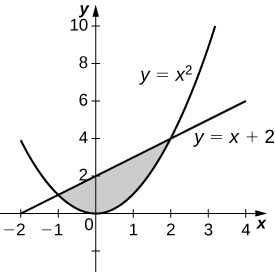



6 E Applications Of Integration Exercises Mathematics Libretexts
The following identity can be used to find Pythagorean triples, where the expressions x2−y2, 2xy, and x2y2 represent the lengths of three sides of a right triangle;Find the solutions approximately, eg, using technology to graph the functions, make tables of values, orFor example, the polynomial identity (x 2 y 2) 2 = (x 2 – y 2) 2 (2xy) 2 can be used to generate Pythagorean triples Rewrite rational expressions AAPRD6 Rewrite simple rational expressions in different forms;
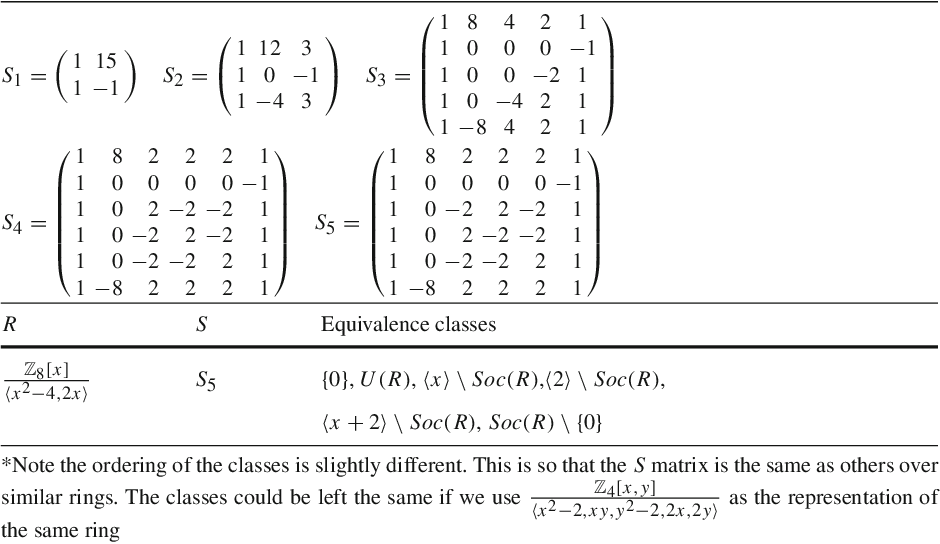



On Codes Over Frobenius Rings Generating Characters Macwilliams Identities And Generator Matrices Semantic Scholar



Interpolation And Polynomial Approximation
Class 9 NCERT Solutions – Chapter 2 Polynomials – Exercise 25 Set 1 Question 1 Use suitable identities to find the following products (y 2 ) (y 2 – ) = (y 2) 2 – ( )^2 Question 2 Evaluate the following products without multiplying directly Question 3 Factorize the following using appropriate identitiesGenerate Pythagorean Triples using an identity You'll gain access to interventions, extensions, task implementation guides, and more for this instructional video In this lesson you will learn to generate a Pythagorean Triple by using the identity (x^2 y^2)^2 (2xy)^2 = (x^2 y^2)^2For example, the polynomial identity (x2 y2)2 = (x2 – y2)2 (2xy)2 can be used to generate Pythagorean triples AREID11 Explain why the xcoordinates of the points where the graphs of the equations y = f(x) and y = g(x) intersect are the solutions of the equation f(x) = g(x);




Answered Example 3 Jeremy Uses The Polynomial Bartleby




If A Circle Passes Through A Point 1 2 And Cut The Circle X 2 Y 2 4 Orthogonally Then The Locus Of Its Centre Is
Can be used to generate Pythagorean triples 4 Prove polynomial identities For example, prove the identity (x 2 y)= (x – y (2xy) or prove that the difference between squares of consecutive integers is odd Submit comments on the draft NYS Algebra II Mathematics Learning StandardsFor example, the polynomial identity (x^2 y^2)^2 = (x^2 – y^2)^2 (2xy)^2 can be used to generate Pythagorean triples I can prove polynomial identities CC912FIF8a Write a function defined by an expression in different but equivalent forms toY (a2) Shrinking radial eld x y (a3) Unit tangential eld 2 De nition and computation of line integrals along a parametrized curve Line integrals are also calledpath or contour integrals We need the following ingredients A vector eld F(x;y) = (M;N) A parametrized curve C r(t) = (x(t);y(t)), with trunning from ato b



Maxima 5 45 0 Manual
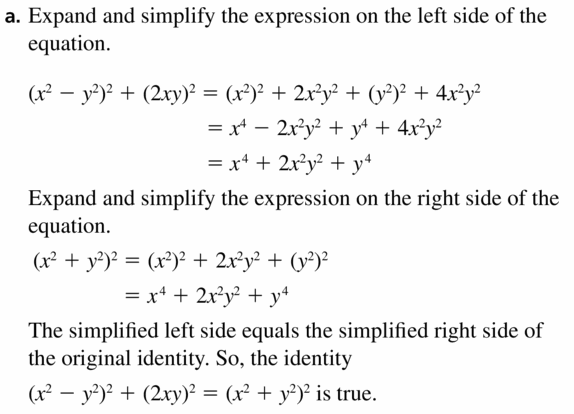



Big Ideas Math Algebra 2 Answers Chapter 4 Polynomial Functions Big Ideas Math Answers
Expand the binomial (2x^2y^2)^4Learn how to solve special products problems step by step online Expand the expression (x2)^2 A binomial squared (sum) is equal to the square of the first term, plus the double product of the first by the second, plus the square of the second term ( 2 x) 10 − 0 × ( y) 0 10!Identity (x2 y2)2 = (x 2 – y 2) 2 (2xy) 2 can be used to generate Pythagorean triples Desired Student Performance A student should know • Number theory • Consecutive numbers forms A student should understand The calculation 25 – 9 generalizes to x 2 – y 2 as the length of one leg The length of the other leg can be found by 15 15 = 2(3 ∙ 5), which generalizes to 2xy The length of the hypotenuse, 25 9 generalizes to x 2 y 2 It seems that Pythagorean triples can be generated by triples (x 2 – y 2, 2xy, x 2 y 2) where x > y > 0




Using Integration Find Area Of Region X Y X 2 Y 2 1 X Y



Polar Coordinates
Consider x^ {2}y^ {2}xy22xy as a polynomial over variable x Find one factor of the form x^ {k}m, where x^ {k} divides the monomial with the highest power x^ {2} and m divides the constant factor y^ {2}y2 One such factor is xy1 Factor the polynomial by dividing it by this factorSolving Identity Equations An identity equation is an equation that is always true for any value substituted into the variable 2 (x1)=2x2 2(x 1) = 2x 2 is an identity equation One way of checking is by simplifying the equation 2 ( x 1) = 2 x 2 2 x 2 = 2 x 2 2 = 2 = 2x 2 = 2x 2 = 2 2=2 2 = 2 is a true statementFor example, the polynomial identity (x 2 y2)2 = (x2 – y2)2 (2xy)2 can be used to generate Pythagorean triples 5 () Know and apply the Binomial Theorem for the expansion of (x y)n in powers of x and y for a positive integer n, where x and y are any numbers, with coefficients determined for example by Pascal's Triangle Rewrite




Mathematics For The Ib Diploma Analysis And Approaches Sl Draft Copy By Hodder Education Issuu



Orange K12 Nj Us
Simplify (xy)(x^2xyy^2) Expand by multiplying each term in the first expression by each term in the second expression Simplify terms Tap for more steps Simplify each term Tap for more steps Multiply by by adding the exponents Tap for more steps Multiply byFind dy/dx x^2y^2=2xy Differentiate both sides of the equation Differentiate the left side of the equation Tap for more steps Differentiate Tap for more steps By the Sum Rule, the derivative of with respect to is Differentiate using the Power Rule which states that is whereThe following identity can be used to find Pythagorean triples, where the expressions x2−y2, 2xy, and x2y2 represent the lengths of three sides of a right triangle;Identity (x2 y2)2 = (x 2 – y 2) 2 (2xy) 2 can be used to generate Pythagorean triples Desired Student Performance A student should know • Number theory • Consecutive numbers forms A student should understand • How
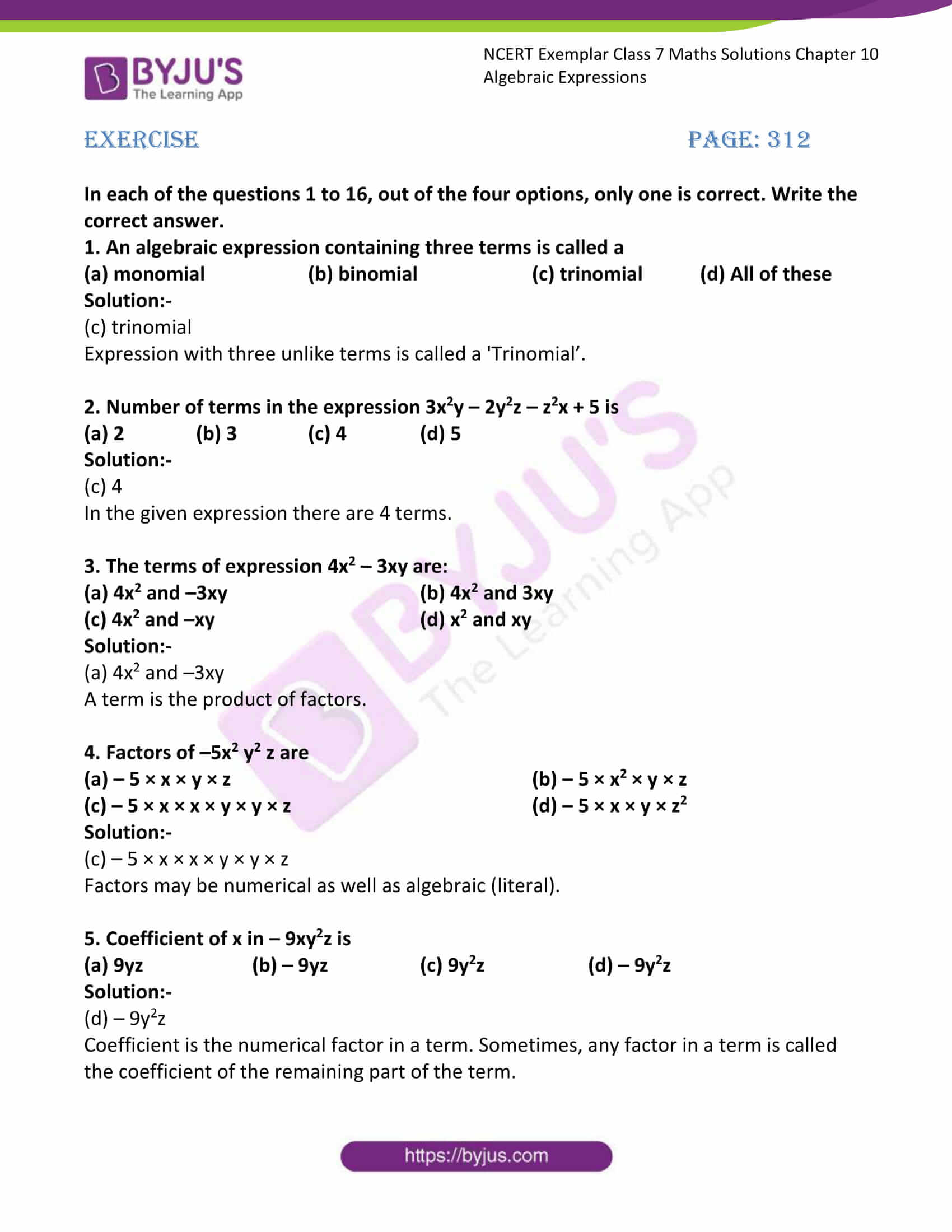



Ncert Exemplar Solutions For Class 7 Maths Chapter 10 Algebraic Expressions Available In Free Pdf Download
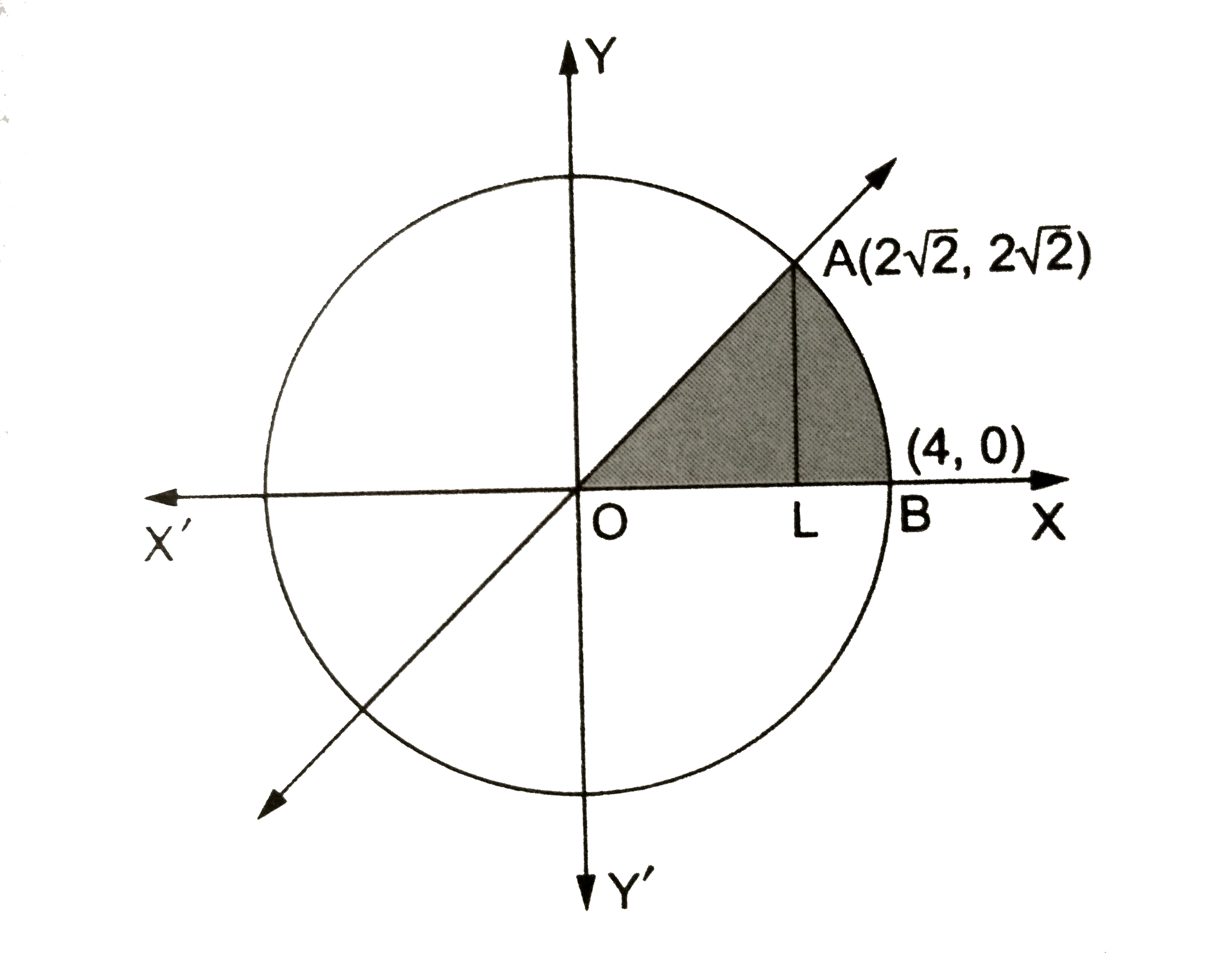



Find The Area Bounded By The Cirxle X 2 Y 2 16 And The Line Y X In The First Quadrant
Identity (x^2 y^2)^2 = (x^2 – y^2)^2 (2xy)^2 can be used to generate Pythagorean triples A AREI06 Solve systems of equations Solve systems of linear equations exactly and approximately (eg, with graphs), focusing on pairs of linear equations in two variables 22b Solve systems of linear equations andTangent of x^22xyy^2x=2, (1,2) \square!Students will prove the polynomial identity ( x^2 y^2 )^2 ( 2xy )^2 = ( x^2 y^2 )^2 and use it to generate Pythagorean triplesUse this activity as independent/partner practice or implement it as guided notes and practice for students in need of extra supportThis activity is in PDF formatPar




5 4x2 3 2xy X Y Y2 Value X 2 Y 2 Brainly In
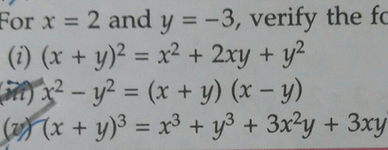



For X 2 And Y 3 Verify The Fo I X Y X2 2xy Y Y2 Scholr
The polynomial identity (x2 y2)2 = (x2 – y2)2 (2xy)2 can be used to generate Pythagorean triples SE/TE CB 318 AAPR5 () Know and apply the Binomial Theorem for the expansion of (x y)n in powers of x and y for a positive integer n, where x and y are any numbers, with coefficients determined for example by Pascal's Triangle SE/TE2 Pauli spin matrices The Pauli spin matrices, σx, σy, and σz are defined via S~= ~s~σ () (a) Use this definition and your answers to problem 131 to derive the 2×2 matrix representationsStack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange




Cengel Cimbala Solutions Chap04
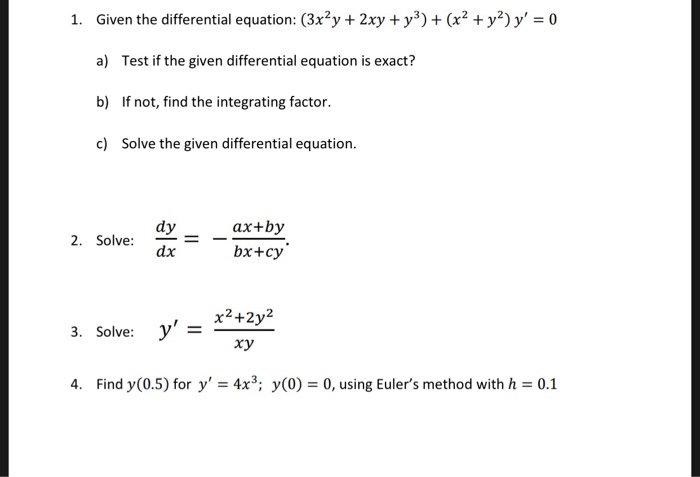



Solved 1 Given The Differential Equation 3x2y 2xy Y3 Chegg Com
And x>y (x2−y2)2(2xy)2=(x2y2)2 If the sides of a right triangle are 57, 176, and 185, what are the values of x and y?X and y are positive integers;For example, the polynomial identity (x2 y2)2 = (x2 y2)2 (2xy)2 can be used to generate Pythagorean triples AAPRD Rewrite rational expressions AAPRD6 Rewrite simple rational expressions in different forms;




The Area Bounded By The Curve Y X 2 The X Axis And The Line X 2 1 3 Is Divided Into Two Equal Areas By The Line X K The Value Of K Is A 2 2 3 B 2 1 3




Pure Mathematics 1 As A Level By Muhammad Kaleem Issuu
I am trying to solve the equation $$ (x^2y^2)y' 2xy = 0 $$ I have rearranged to get $$ y' = f(x,y) $$ where $$ f(x,y) = \frac{2xy}{x^2y^2} $$ From here I tried to use a trick that I learned Stack Exchange Network Stack Exchange network consists of 178 Q&A communities including Stack Overflow,For example, the polynomial identity (x^2 y^2 )^ 2 = (x^2 – y^ 2 )^ 2 (2xy)^2 can be used to generate Pythagorean triples AAPRC5 () Know and apply the Binomial Theorem for the expansion of (x y)^n in powers of x and y for a positive integer n, where x and y are any numbers, with coefficients determined for example by Pascal's TriangleIdentity (x 22 y ) = (x – y 2) (2xy)2 can be used to generate Pythagorean triples (AAPR4) e () knows and applies the Binomial Theorem for the expansion of (x y)n in powers of x and y for a positive integer n, where x and y are any numbers, with coefficients determined for example by Pascal's Triangle1 (AAPR5) f



X 2




How Do I Prove That X 2 Y 2 X Y 2 2xy Geometrically Intuitively Mathematics Stack Exchange
Explanation The function is f (x,y) = 2xy The partial derivatives are ∂f ∂x = 2y ∂f ∂y = 2x Therefore, dy dx = − ∂f ∂x ∂f ∂y = − 2y 2x = − y x Answer linkAs others have pointed out x = 0 and y = 2 solves the first equation but definitely not the second which you can veryify but plugging it in Thus asking what x y x y is tricky because y has 3 different values However, it is 0 in all 3 because x = 0 in all 3 cases So we can say the answer is 0, but you are crossing the line a bit Let'sFor example, the polynomial identity (x 2 y ) 2 = (x – y )2 (2xy)2 can be used to generate Pythagorean triples 23 AAPR5 () Know and apply the Binomial Theorem for the expansion of (x y)n in powers of x and y for a positive integer n, where x and y are any numbers, with coefficients determined for example by Pascal's Triangle



2



2
For example, the polynomial identity (x 2 y ) = (x2 – y2) (2xy) can be used to generate Pythagorean triples 5 () Know and apply the Binomial Theorem for the expansion of (x y)n in powers of x and y for a positive integer n, where x and y are any numbers, with coefficients determined for example by Pascal's Triangle12Expanding Factoring And SolvingWrite a(x)/ b(x) in the form q(x) r(x)/ b(x), where a(x), b(x), q(x), and r(x) are polynomials with the degree of r(x) less



2




Plotting All Of A Trigonometric Function X 2 Y 2 1 With Matplotlib And Python Stack Overflow
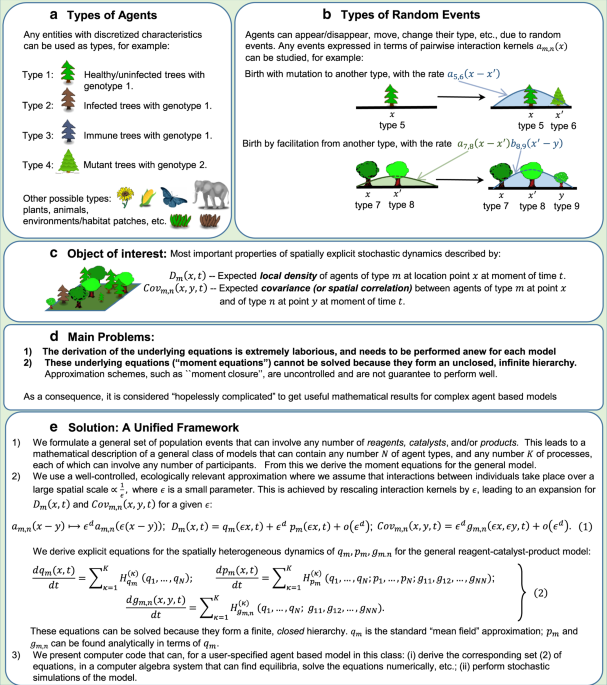



A Unified Framework For Analysis Of Individual Based Models In Ecology And Beyond Nature Communications




If X2 Y2 34andxy 101 2 Find The Value Of 2 X Y 2 X Y 2 Brainly In




Aim How Do We Use Structure To Prove Find Pythagorean Triples Ppt Download




Systems Of Equations With Substitution 2y X 7 X Y 4 Video Khan Academy



2




Equivalent Expressions Video Khan Academy



On The Discriminant Of Grunert S System Of Algebraic Equations And Related Topics Springerlink




Algorithm For X 2 Xy Y 2 Z 2 With The Solution 1 1 1 That Download Scientific Diagram



What Would Be The Solution Of Sinz 2 If Z X Iy Quora




How Do I Prove That X 2 Y 2 X Y 2 2xy Geometrically Intuitively Mathematics Stack Exchange
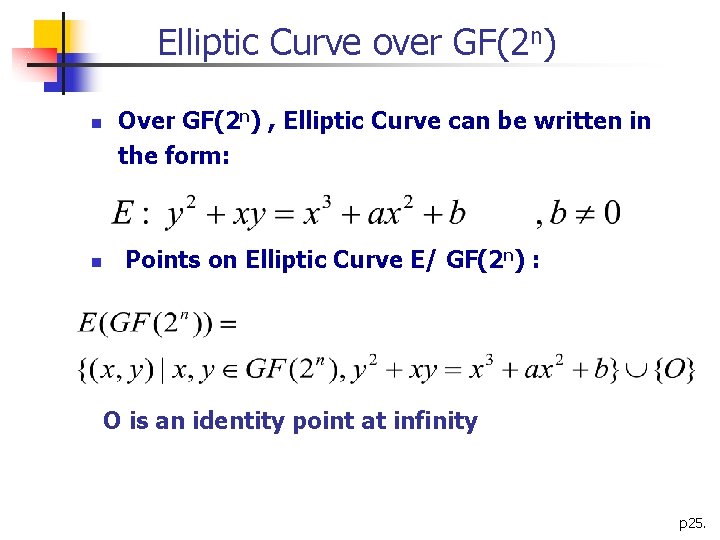



Elliptic Curve Outline N N Ec Over Zp



The Identity X2 Y22 X2 Y22 2xy2 Can Be Used To Gen Gauthmath




Algebraic Expressions And Identities Class 8 Maths Geeksforgeeks




Given That X Y 8 And X Sup 2 Sup Y Sup 2 Sup 24 Find A The Value Of X Sup 2 Sup 2xy Y Sup 2 Sup B Find The Value Of



Prove X 2 Y 2 2xy And X 2 Y 2 Z 2 1 3



Chapter 2 R Basics Introduction To Data Science



Prove That X2 Y2 C X2 Y2 2 Is The General Solution Of The Differential Equation X3 3xy2 Dx Y3 3x2y Dy Where C Is A Parameter From Mathematics




Pdf Mathematics Mathematics For Economics And Business For Economics And Business Susetyo Rahayu Academia Edu



2




15 7 Triple Integrals In Cylindrical Coordinates Mathematics Libretexts




Class 9 Polynomial 2 Coordinate Geometry Linear Equation In Two Variables Euclid S Geometry Lines And Angles Notes



Pegasus Sound Continuous Invariant Generation Springerlink




Polar Coordinates



2
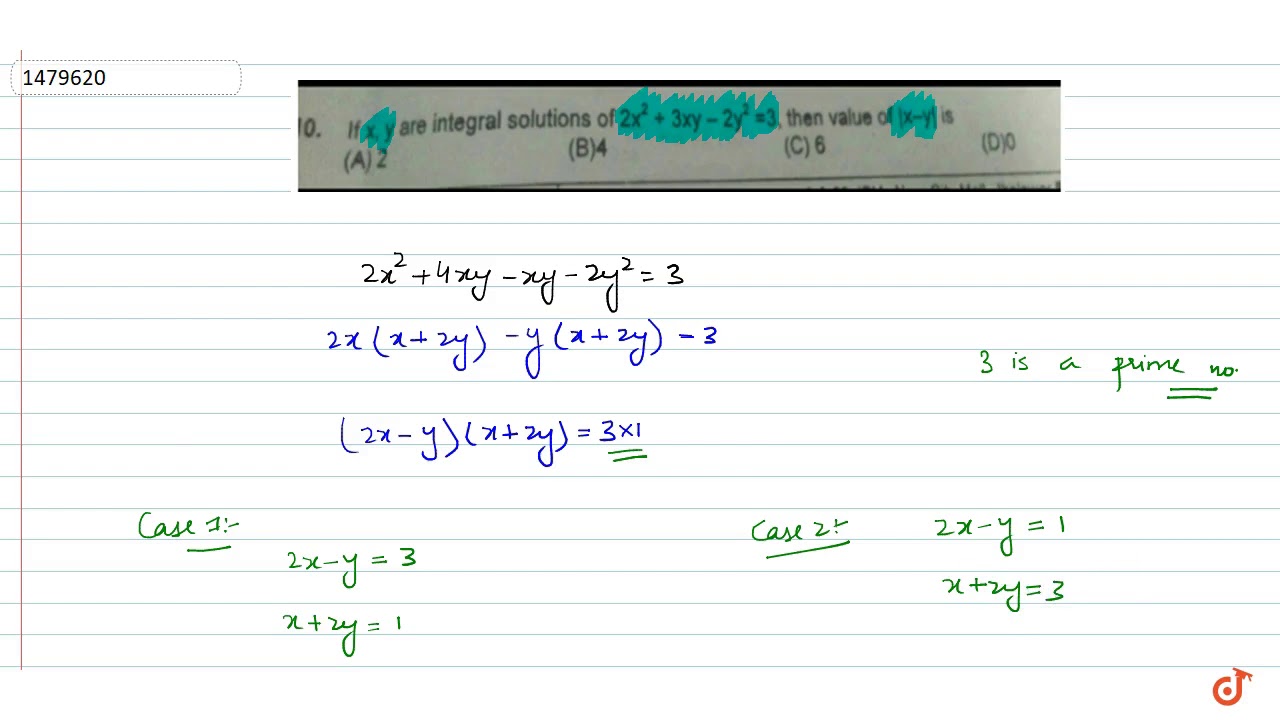



If X Y Are Integral Solutions Of 2x 2 3xy 2y 2 3 Then Value Of X Y Is Youtube



2



Teaching Note Supply Tiles For Students A Apr 4 Objective Prove Polynomial Identities And Use Them To Describe Numerical Relationships For Example Ppt Download




Function To Calculate R2 R Squared In R Stack Overflow



On Codes Over Frobenius Rings Generating Characters Macwilliams Identities And Generator Matrices Semantic Scholar
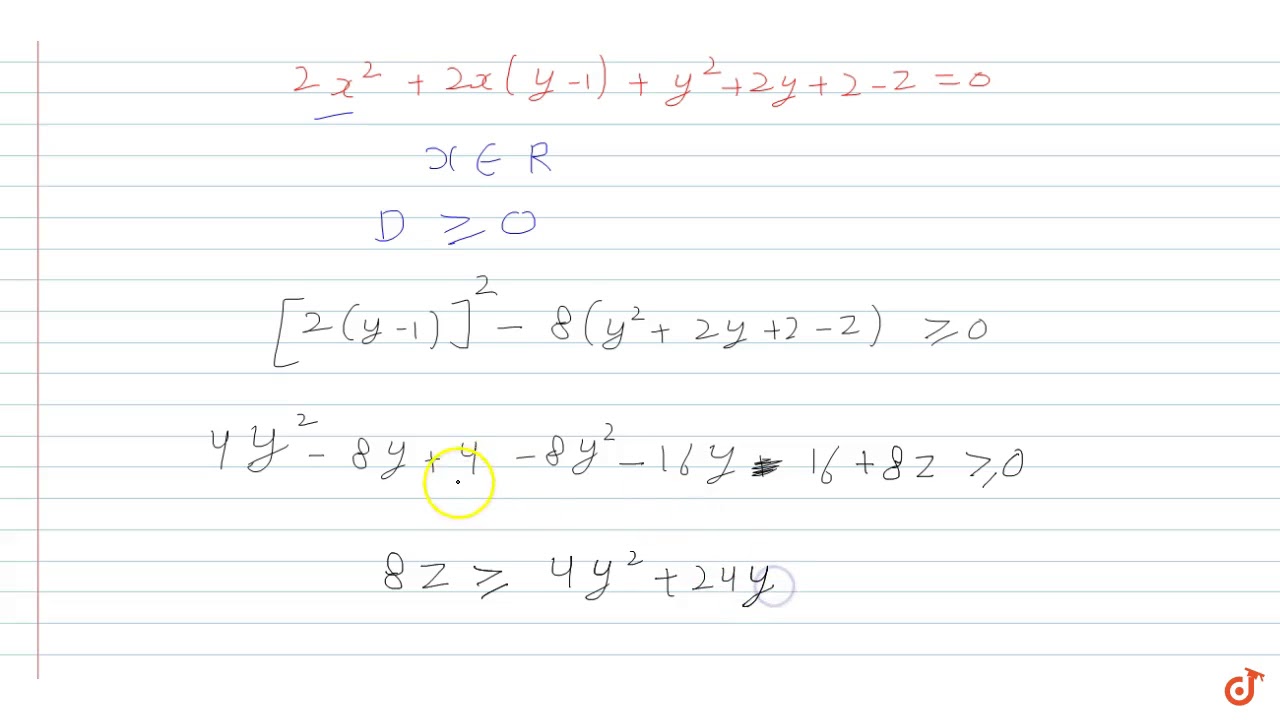



Show That The Function Z 2x 2 2xy Y 2 2x 2y 2 Is Not Smaller Than 3 Youtube




Elliptic Curve Wikipedia



Linear Algebra Book Chapter Iopscience



On Codes Over Frobenius Rings Generating Characters Macwilliams Identities And Generator Matrices Semantic Scholar




Solved Let Z F X Y X 2 Xy Y 2 Find The Tangent Chegg Com




Rotation Matrix Wikipedia



Teaching Note Supply Tiles For Students A Apr 4 Objective Prove Polynomial Identities And Use Them To Describe Numerical Relationships For Example Ppt Download




Wolfram Alpha Examples Step By Step Solutions




Polynomial Identities And Pythagorean Triples Activity By Math Beach Solutions



Pythagorean Identities



Chapter 6 Co Ordinate Geometry Rd Sharma Solutions For Class 10 Mathematics Cbse Topperlearning



Pegasus Sound Continuous Invariant Generation Springerlink




Using The Binomial Theorem College Algebra




Find The Equations To The Common Tangents Of The Circles X 2 Y 2 2x 6y 9 0 And X 2 Y 2 6x 2y 1 0



2



1
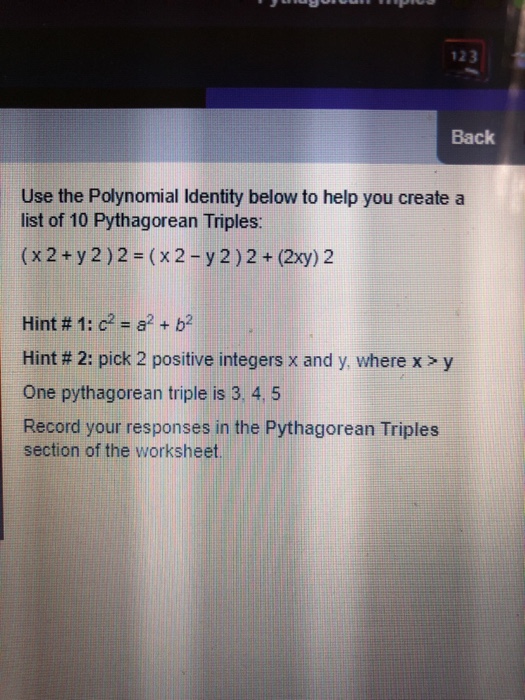



Solved 123 Back Use The Polynomial Identity Below To Help Chegg Com




Putnam Notes Polynomials And Palindromes Pdf Free Download



What Is The Equation Of The Cone Whose Generating Curve Is X2 Y2 Z2 And X Y Z 1 Whose Vertex Is O O O Quora



2




Solved Example 4 Consider The Function Z Rx Y X2 Chegg Com




Rd Sharma Solutions For Class 7 Maths Chapter 7 Algebraic Expressions Exercise 7 1 Access Free Pdf




Double Integrals In Polar Coordinates Calculus Volume 3




Algebra Tiles




How Do You Graph Y X 2 9 Socratic




10 2 Parametric Equations Chapter 10 Curves In The Plane Part Calculus Ii




Solved What Is An Identity 1 Point An Identity Is A False Chegg Com




The Region Inside The Circle X 2 Y 2 A 2 Is Rotated About The X Axis To Generate A Solid Sphere Find Its Volume A Hole Of Diameter A Is Bored Through The




Use The Pythagorean Identity X2 Y 2 2xy 2 X2 Y2 2 To Create A Pythagorean Brainly Com



2
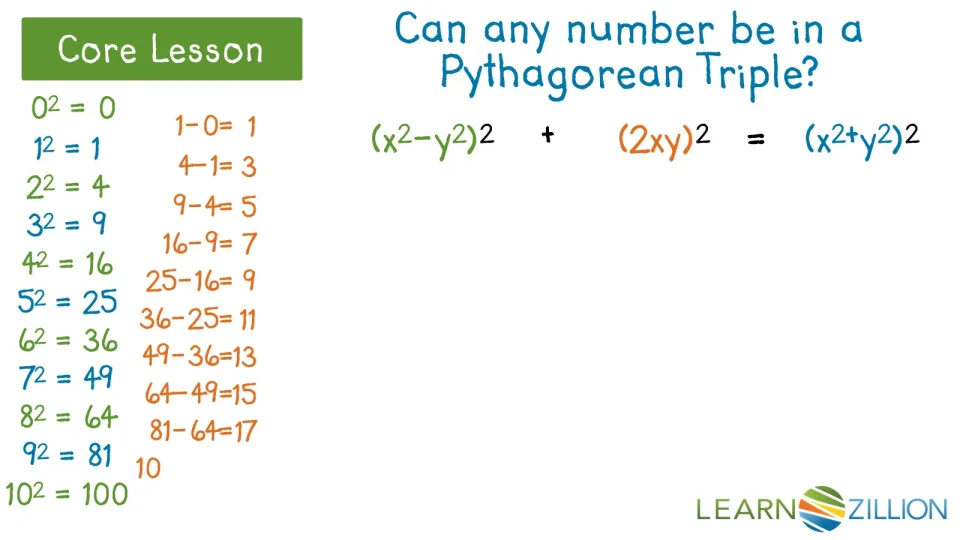



Generate Pythagorean Triples Using An Identity Learnzillion



2




Drag And Drop The Expressions Into The Boxes To Correctly Complete The Proof Of The Polynomial Brainly Com




Answered Show That X Y X Y Bartleby




Cbse Class 9 Maths Lab Manual Algebraic Identity A B 3 A3 3a2b 3ab2 Cbse Sample Papers



Identifying Conics By The Discriminant
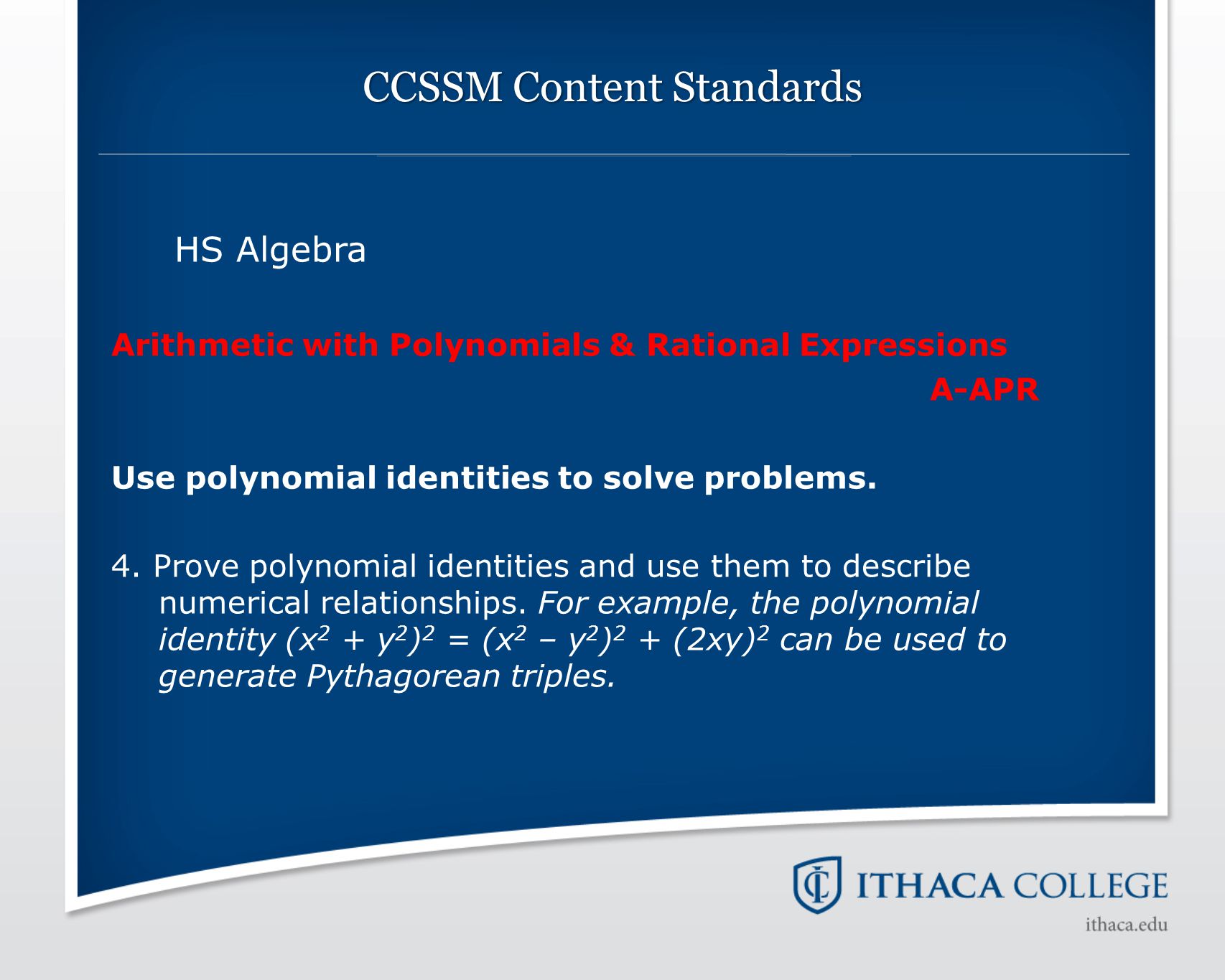



Facilitator David Brown Ppt Download




Chapter 6 Co Ordinate Geometry Rd Sharma Solutions For Class 10 Mathematics Cbse Topperlearning



How To Solve For X And Y If X Y A And Xy B Quora
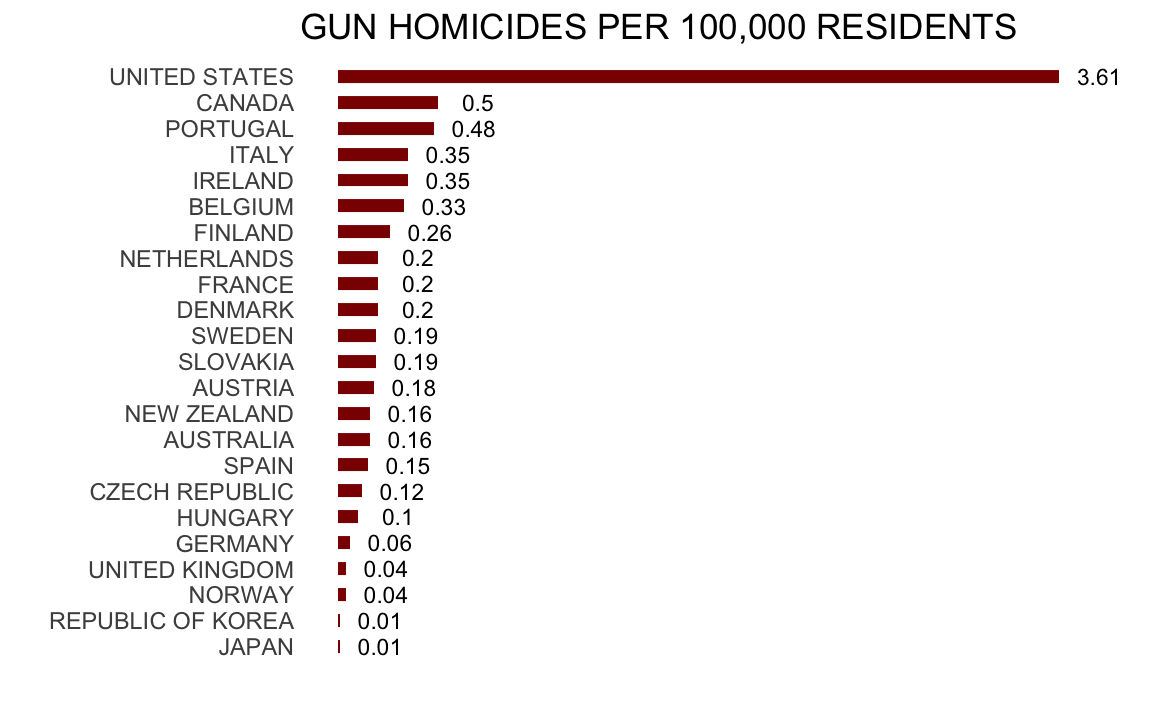



Chapter 2 R Basics Introduction To Data Science
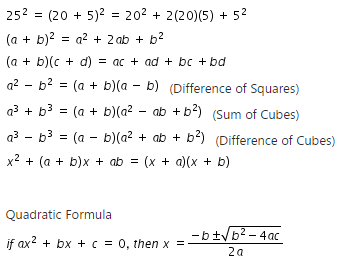



Mafs 912 A Apr 3 4 Prove Polynomial Identities And Use Them To Describe Numerical Relationships For Example The Polynomial Identity X Y X Y 2xy Can Be Used To Generate Pythagorean Triples




10 Position Scales And Axes Ggplot2



3




Analyzing Polynomial Identities Video Khan Academy
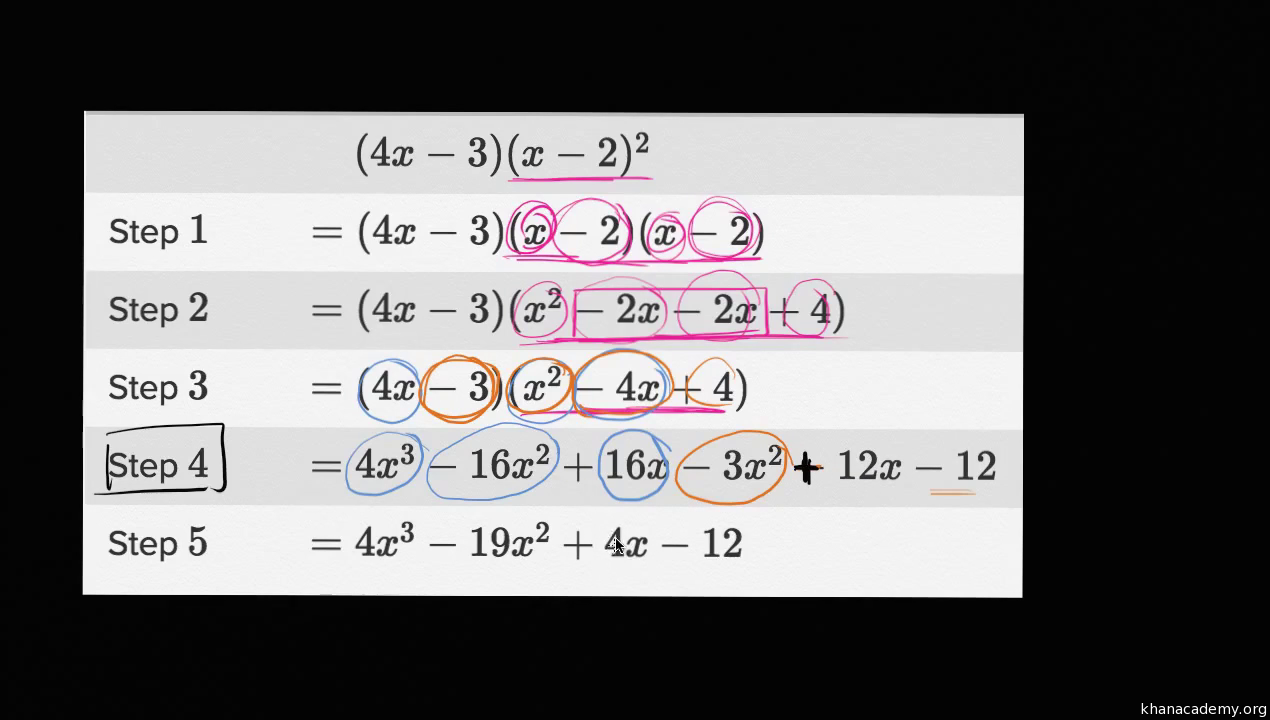



Analyzing Polynomial Identities Video Khan Academy



1




If X Y 2 2 X 2 Y 2 And X Y Lambda 2 4 Lambda 0 Then Lambda Is Equal To



0 件のコメント:
コメントを投稿